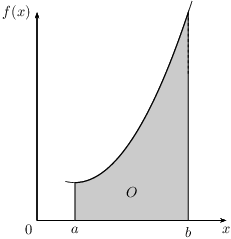
From the theorem on integrals and areas it follows that there is a relation between the integral and the area of a region below/above the graph of a function. We discuss this relation in more detail. Consider the area $O(f,a,b)$ of the region enclosed by the graph of the function $f(x)$, the $x$-axis and the lines $x=a$ and $x=b$, shown in the following figure.
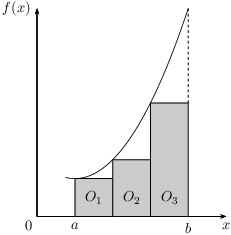
In general it is not easy to calculate the area when $f(x)$ is non-linear. We can provide an approximation for the area by splitting the interval $[a,b]$ up into smaller subintervals. For each subinterval we determine the area of the bar that best fits below the graph of $f(x)$. When we sum the areas of these bars we obtain a reasonable lower-bound for the area of $O(f,a,b)$. In the following figure we use for instance three bars to approximate the area $O(f,a,b)$, hence, $O(f,a,b)\approx O_1+O_2+O_3$.
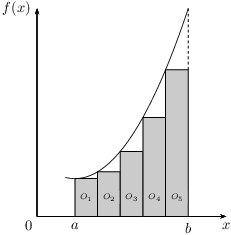
By increasing the number of subintervals we improve the approximation. In the following figure we use five bars to approximate the area of $O(f,a,b)$, hence $O(f,a,b)\approx O_1+O_2+O_3+O_4+O_5$.
If the number of bars becomes infinite, then the approximation becomes exact and we can replace the $\approx$-sign by a $=$-sign. Hence, $O(f,a,b)=O_1+O_2+\ldots+O_n$, with $n$ an infinitely large number.

In general it is not easy to calculate the area when $f(x)$ is non-linear. We can provide an approximation for the area by splitting the interval $[a,b]$ up into smaller subintervals. For each subinterval we determine the area of the bar that best fits below the graph of $f(x)$. When we sum the areas of these bars we obtain a reasonable lower-bound for the area of $O(f,a,b)$. In the following figure we use for instance three bars to approximate the area $O(f,a,b)$, hence, $O(f,a,b)\approx O_1+O_2+O_3$.

By increasing the number of subintervals we improve the approximation. In the following figure we use five bars to approximate the area of $O(f,a,b)$, hence $O(f,a,b)\approx O_1+O_2+O_3+O_4+O_5$.

If the number of bars becomes infinite, then the approximation becomes exact and we can replace the $\approx$-sign by a $=$-sign. Hence, $O(f,a,b)=O_1+O_2+\ldots+O_n$, with $n$ an infinitely large number.

